Quadratic Equations
- Lavya Patel
- Aug 8, 2020
- 3 min read
It always comes back…

It is everywhere… You just have to look for it
What is a Quadratic Trend?
With a quadratic trend, the values of the 'y' values tend to rise or fall at a rate that is not constant; it changes over time, and at each consecutive 'x' values, the rate of change will be different.. As a result, the trend is not a straight line.
The Standard form -
Just like any other model, it will always have an input, once equated, will give us the output -
f(x) = ax² + bx + c or ax² + bx + c = 0
Where ;
a = The value of a determines how steep or broad the curve will look like. The higher the value, the steeper the curve, and the lesser the value, the broader the slope. Another impact of the value of a has on the graph, is that it determines whether the slope is concave up (if the value of a is positive), or concave down (if the value is negative).
x = The input
c = Y - Intercept ; occurs when, x = 0
b = a constant
Things we can find from the equation -
f(x) = ax² + bx + c
f(x) = -2x² + 3x + 6
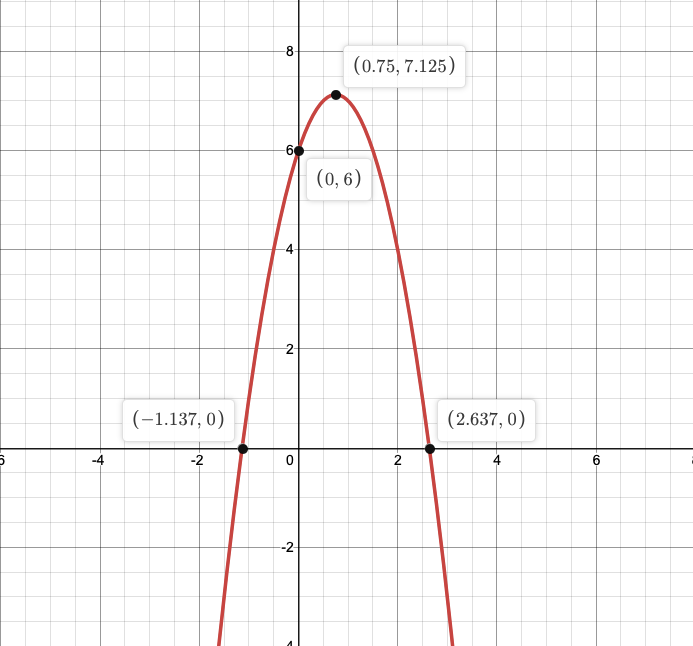
1. The vertex / the maximum and the minimum point
The vertex will always be on the line of symmetry, and that is where the vertex occurs. In this case, since the value of a is -2, the graph of this function will be concave down.
Line of Symmetry Formula - - b / 2a
∴ - 3 / -2(-2) = 3/4 = 0.75
∴ x = 0.75 Is the line of symmetry
2. The y-intercept of the function
Y - intercept is also equal to the value of c
Since y axis occurs when x = 0
f( x = 0) = -2(0)² + 3(0) + 6
∴ f( x = 0) = 6 ; y Intercept = 6 ; c = 6
3. The zeroes of the function
The zeroes of the function are the x intercepts of the graph, therefore, occurs when y = 0
There are 3 possibilities -

There can either be 2 imaginary roots, there can be 2 different roots, of 2 same roots for all quadratic functions.
There are 2 ways the zeroes of the function can be calculated.
Factorisation
Factorisation, also known as splitting the middle term. This is a way of obtaining the roots of the graph f(x) just be slipping the middle term, which is bx. In this method the coefficient of x is spliced into 2 numbers, considering the sum of these 2 numbers is the value of c.
Using the Quadratic Function
With the quadratic function, we can get the 2 roots of the function just by substituting the values into the quadratic formula.

Substituting the constants into this formula and evaluating them would give us the 2 roots of the quadratic function.
With these three findings we can plot a graph for f(x) = -2x² + 3x + 6
There are numerous ways we can write the quadratic function -
1. The standard Form -
f(x) = ax² + bx + c or ax² + bx + c = 0
2. Factorised Form
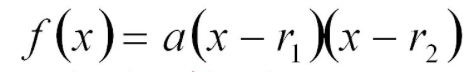
Where the inverse of r1 and r2 are equal to the roots.
3. Vertex Form -
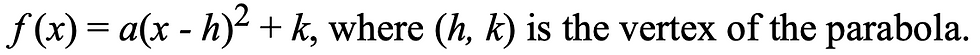


Comments